
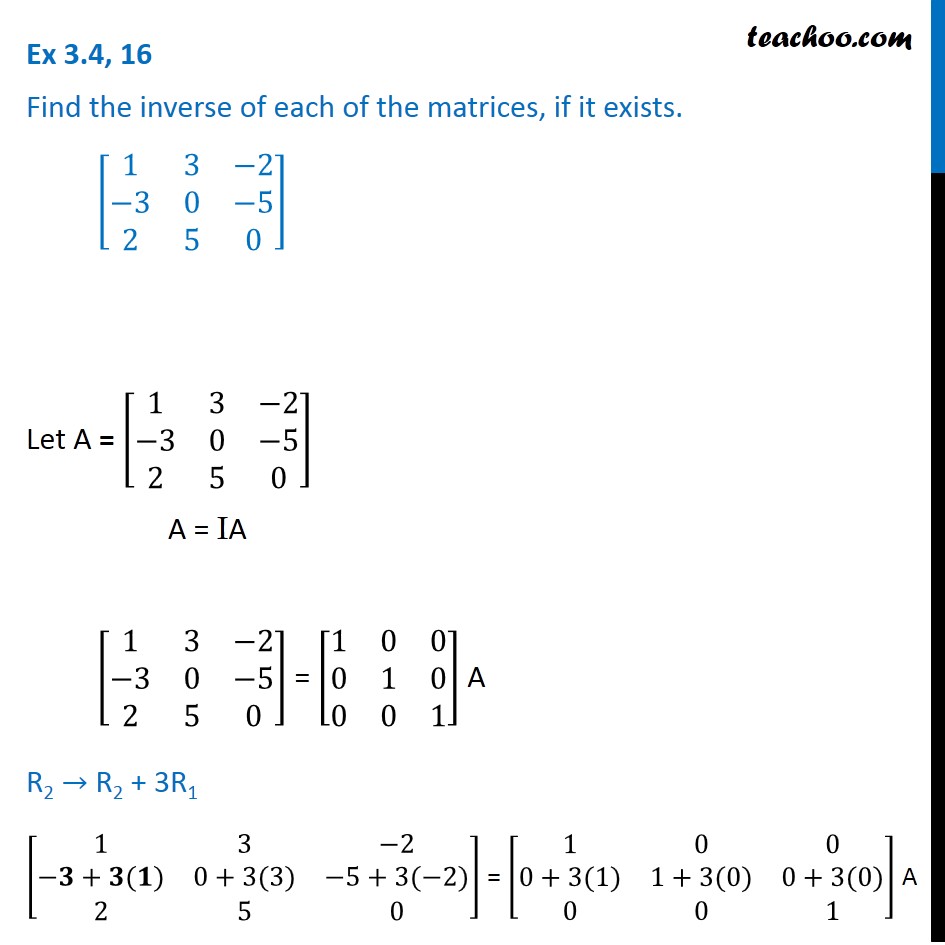
The identity matrix I n is a n x n square matrix with the main diagonal of 1’s and all other elements are O’s. The identity matrix of n*n is represented in the figure below Make sure to perform the same operations on RHS so that you get I=AB. Validate the sum by performing the necessary column operations on LHS to get I in LHS. Write A = AI, where I is the identity matrix as order as A.Ģ. If A -1 exists then to find A -1 using elementary column operations is as follows:ġ. Make sure to perform the same operations on RHS so that you get I=BA. Validate the sum by performing the necessary row operations on LHS to get I in LHS. Write A = IA, where I is the identity matrix as order as A.Ģ. If A -1 exists then to find A -1 using elementary row operations is as follows:ġ.

To find out the required identity matrix we find out using elementary operations and reduce to an identity matrix Let us take 3 matrices X, A, and B such that X = AB. In this method first, write A=IA if you are considering row operations, and A=AI if you are considering column operation. This method is suitable to find the inverse of the n*n matrix.
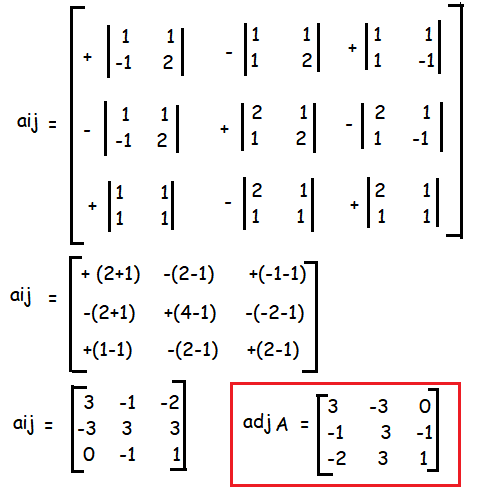
Where adj ( A ) refers to the adjoint matrix A, |A| refers to the determinant of a matrix A.Īdjoint of a matrix is found by taking the transpose of the cofactor matrix.Ĭ ij = (-1) ij det (Mij), C ij is the cofactor matrix
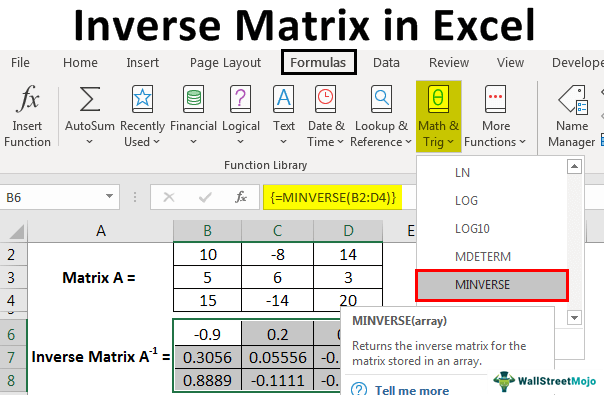
This matrix inversion method is suitable to find the inverse of the 2 by 2 matrix. If A is symmetric then its inverse is also symmetric.īroadly there are two ways to find the inverse of a matrix: If A and B are invertible then (AB) -1 = B -1 A -1 The inverse of a square matrix, if exists, is unique
#Inverse matrix how to#
A common question arises, how to find the inverse of a square matrix? By inverse matrix definition in math, we can only find inverses in square matrices. The square matrix has to be non-singular, i.e, its determinant has to be non-zero. How to find the inverse of a matrix/ how to determine the inverse of a matrix? The inverse matrix can be found only with the square matrix. Image will be uploaded soon Rank of The Matrix - The rank of the matrix is the extreme number of linearly self-determining column vectors within the matrix. According to the inverse of a matrix definition, a square matrix A of order n is said to be invertible if there exists another square matrix B of order n such that AB = BA = I. Let us first define the inverse of a matrix. In that, most weightage is given to inverse matrix problems.
12.2.2 Determinants: terminology required for the general formula.12.2.1 Determinants: formulas for small sizes.Exercises 10 (Arithmetic and geometric progressions).10 Arithmetic and geometric progressions.Exercises 8 (Long division and the Remainder theorem).8.2 Obtaining the factorisation of a polynomial.3.1 General formula for roots of \(ax^2+bx+c\).1.2 Solving a pair of linear equations in two variables.


 0 kommentar(er)
0 kommentar(er)
